This article relies largely or entirely on a single source. (August 2021) |
In mathematics, the polygamma function of order m is a meromorphic function on the complex numbers defined as the (m + 1)th derivative of the logarithm of the gamma function:

Thus
holds where ψ(z) is the digamma function and Γ(z) is the gamma function. They are holomorphic on . At all the nonpositive integers these polygamma functions have a pole of order m + 1. The function ψ(1)(z) is sometimes called the trigamma function.
 |
 |
 |
| ln Γ(z) | ψ(0)(z) | ψ(1)(z) |
 |
 |
 |
| ψ(2)(z) | ψ(3)(z) | ψ(4)(z) |
Integral representation edit
When m > 0 and Re z > 0, the polygamma function equals
where is the Hurwitz zeta function.
This expresses the polygamma function as the Laplace transform of (−1)m+1 tm/1 − e−t. It follows from Bernstein's theorem on monotone functions that, for m > 0 and x real and non-negative, (−1)m+1 ψ(m)(x) is a completely monotone function.
Setting m = 0 in the above formula does not give an integral representation of the digamma function. The digamma function has an integral representation, due to Gauss, which is similar to the m = 0 case above but which has an extra term e−t/t.
Recurrence relation edit
It satisfies the recurrence relation
which – considered for positive integer argument – leads to a presentation of the sum of reciprocals of the powers of the natural numbers:
and
for all , where is the Euler–Mascheroni constant. Like the log-gamma function, the polygamma functions can be generalized from the domain uniquely to positive real numbers only due to their recurrence relation and one given function-value, say ψ(m)(1), except in the case m = 0 where the additional condition of strict monotonicity on is still needed. This is a trivial consequence of the Bohr–Mollerup theorem for the gamma function where strictly logarithmic convexity on is demanded additionally. The case m = 0 must be treated differently because ψ(0) is not normalizable at infinity (the sum of the reciprocals doesn't converge).
Reflection relation edit
where Pm is alternately an odd or even polynomial of degree |m − 1| with integer coefficients and leading coefficient (−1)m⌈2m − 1⌉. They obey the recursion equation
Multiplication theorem edit
The multiplication theorem gives
and
for the digamma function.
Series representation edit
The polygamma function has the series representation
which holds for integer values of m > 0 and any complex z not equal to a negative integer. This representation can be written more compactly in terms of the Hurwitz zeta function as
This relation can for example be used to compute the special values[1]
Alternately, the Hurwitz zeta can be understood to generalize the polygamma to arbitrary, non-integer order.
One more series may be permitted for the polygamma functions. As given by Schlömilch,
This is a result of the Weierstrass factorization theorem. Thus, the gamma function may now be defined as:
Now, the natural logarithm of the gamma function is easily representable:
Finally, we arrive at a summation representation for the polygamma function:
Where δn0 is the Kronecker delta.
Also the Lerch transcendent
can be denoted in terms of polygamma function
Taylor series edit
The Taylor series at z = -1 is
and
which converges for |z| < 1. Here, ζ is the Riemann zeta function. This series is easily derived from the corresponding Taylor series for the Hurwitz zeta function. This series may be used to derive a number of rational zeta series.
Asymptotic expansion edit
These non-converging series can be used to get quickly an approximation value with a certain numeric at-least-precision for large arguments:
and
where we have chosen B1 = 1/2, i.e. the Bernoulli numbers of the second kind.
Inequalities edit
The hyperbolic cotangent satisfies the inequality
and this implies that the function
is non-negative for all m ≥ 1 and t ≥ 0. It follows that the Laplace transform of this function is completely monotone. By the integral representation above, we conclude that
is completely monotone. The convexity inequality et ≥ 1 + t implies that
is non-negative for all m ≥ 1 and t ≥ 0, so a similar Laplace transformation argument yields the complete monotonicity of
Therefore, for all m ≥ 1 and x > 0,
Since both bounds are strictly positive for , we have:
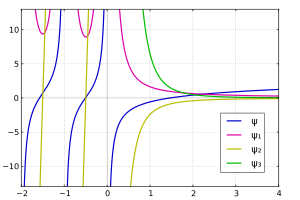
- is strictly convex.
- For , the digamma function, , is strictly monotonic increasing and strictly concave.
- For odd, the polygamma functions, , are strictly positive, strictly monotonic decreasing and strictly convex.
- For even the polygamma functions, , are strictly negative, strictly monotonic increasing and strictly concave.
This can be seen in the first plot above.
Trigamma bounds and asymptote edit
For the case of the trigamma function ( ) the final inequality formula above for , can be rewritten as:
so that for : .
See also edit
References edit
- ^ Kölbig, K. S. (1996). "The polygamma function psi^k(x) for x=1/4 and x=3/4". J. Comput. Appl. Math. 75 (1): 43–46. doi:10.1016/S0377-0427(96)00055-6.
- Abramowitz, Milton; Stegun, Irene A. (1964). "Section 6.4". Handbook of Mathematical Functions. New York: Dover Publications. ISBN 978-0-486-61272-0.



